| The perimeter of a polygon is the distance around the outside of the polygon. A polygon is 2-dimensional; however, perimeter is 1-dimensional and is measured in linear units. To help us make this distinction, look at our picture of a rectangular backyard. The yard is 2-dimensional: it has a length and a width. The amount of fence needed to enclose the backyard (perimeter) is 1-dimensional. The perimeter of this yard is the distance around the outside of the yard, indicated by the red arrow; It is measured in linear units such as feet or meters. | ![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/fence_perimeter.gif) |
 |
| To find the perimeter of a polygon, take the sum of the length of each side. The polygons below are much smaller than a fenced-in yard. Thus, we use smaller units in our examples, such as centimeters and inches. |
 |
|
| Example 1: | Find the perimeter of a triangle with sides measuring 5 centimeters, 9 centimeters and 11 centimeters. | ![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/scalene_triangle.gif) |
| Solution: | P = 5 cm + 9 cm + 11 cm = 25 cm |
|
| Example 2: | A rectangle has a length of 8 centimeters and a width of 3 centimeters. Find the perimeter. | ![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/rectangle_8x3.gif) |
 |
| Solution 1: | P = 8 cm + 8cm + 3 cm + 3 cm = 22 cm |
| Solution 2: | P = 2(8 cm) + 2(3 cm) = 16 cm + 6 cm = 22 cm |
 |
|
In Example 2, the second solution is more commonly used. In fact, in mathematics, we commonly use the following formula for perimeter of a rectangle:
 , where , where  is the perimeter, is the perimeter, 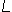 is the length and is the length and  is the width. is the width. |
 |
| In the next few examples, we will find the perimeter of other polygons. |
 |
|
| Example 3: | Find the perimeter of a square with each side measuring 2 inches. | ![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/square_2X2.gif) |
| Solution: |  = 2 in + 2 in + 2 in + 2 in = 8 in = 2 in + 2 in + 2 in + 2 in = 8 in |
|
| Example 4: | Find the perimeter of an equilateral triangle with each side measuring 4 centimeters. | ![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/equilateral_triangle.gif) |
| Solution: |  = 4 cm + 4 cm + 4 cm = 12 cm = 4 cm + 4 cm + 4 cm = 12 cm |

![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/fence_perimeter.gif)
![[IMAGE]](http://www.mathgoodies.com/lessons/vol1/images/scalene_triangle.gif)
No comments:
Post a Comment